Mathematics Introduction, Continuity at a point and Continuity at interval For CBSE-NCERT
Please Wait... While Loading Full Video

class 12 chapter 5 - continuity & Differentiability

Introduction, Continuity at a point and Continuity at interval For CBSE-NCERT

Click for Only Video

Topic covered

`star` Introduction
`star` Continuity at a point
`star`Continuity at interval

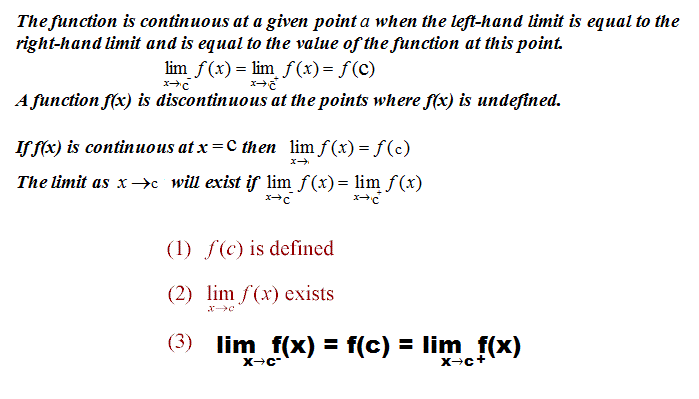
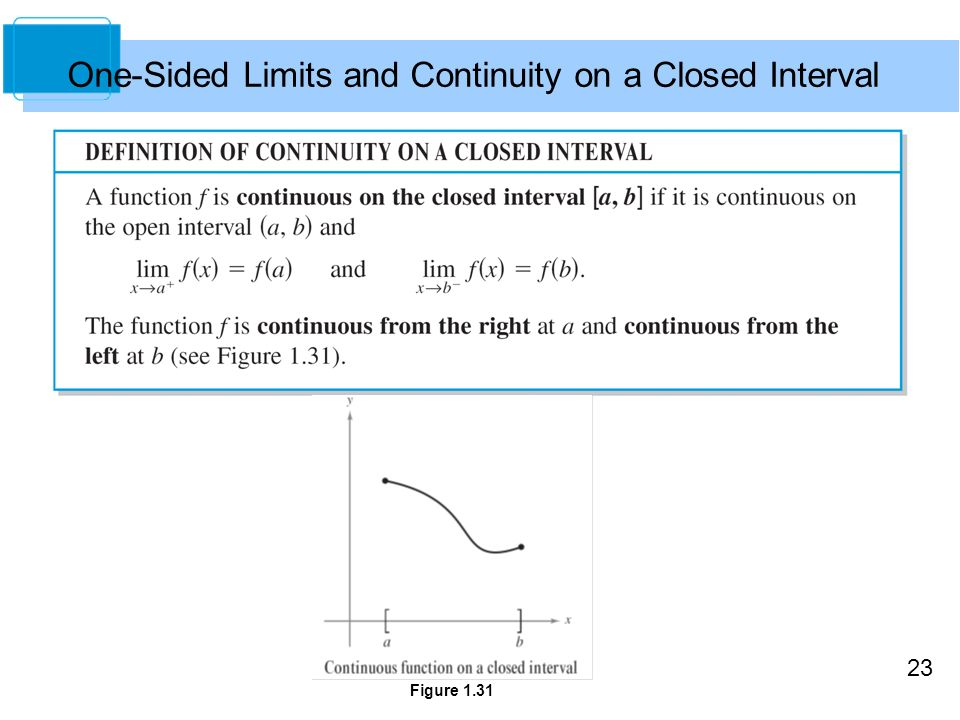
Introduction :

Let's Consider the function

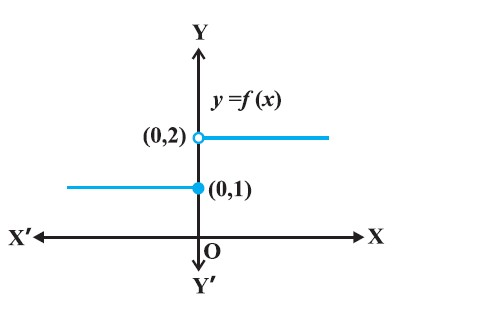
`f(x)= { tt((1, if , x le 0),(2, if , x > 0 ))`

● The value of the function at nearby points on x-axis remain close to each other except at `x = 0.` At the points near and to the left of 0, i.e., at points like `– 0.1, – 0.01, – 0.001,` the value of the function is `1`.

● At the points near and to the right of `0`, i.e., at points like `0.1, 0.01`, 0.001, the value of the function is 2.

● Left (respectively right) hand limit of f at 0 is 1 (respectively 2).

● So here the value of the function at `x = 0` coincides with the left hand limit.

`color{orange}{"Note : we cannot draw this graph in one stroke, i.e., without lifting pen"}`
`color{orange}{" from the plane of the paper ,we need to lift the pen when we come to "}`
`color{orange}{0 \ \ " from left. Means function is not continuous at" x = 0.}`

Continuity At a point

`\color{green} ✍️` If the left hand limit, right hand limit and the value of the function at `x = c` exist and equal to each other, then f is said to be continuous at `x = c`.

`color{orange}{lim_(x->c^-) f(x) =f(c) = lim_(x->c^+) f(x)}`

`"● Note :"` if the right hand and left hand limits at `x = c` coincide, its said the common value is the limit of the function at `x = c`.

● If `f` is not continuous at `c`, we say `f` is discontinuous at `c` and `c` is called a point of discontinuity of `f`.


E.g., Let's examine the function `f` given by `f (x) = x^2` is continuous at `x = 0` or not?

● First note that the function is defined at the given point `x = 0` and its value is `0`.

Then we find the limit of the function at `x = 0`. Clearly



For `c>0` `=>` `lim_(x-> 0^+) f(x) = lim_(x-> 0) x^2=0^2=0`
For `c<0` `=>` `lim_(x-> 0^-) f(x) = lim_(x-> 0) x^2=0^2=0`

● Thus `lim_(x-> 0) f(x) =0 =f(x)`

Hence, `f` is continuous at `x = 0`.

Now let's another example

(ii) E.g., the continuity of the function `f` given by `f(x) = | x |` at `x = 0`.

Here, By definition : `f(x) ={ tt(( -x, if, x < 0),(x, if , x ge 0))`



● Clearly the function is defined at `0` and `f (0) = 0`. Left hand limit of `f` at `0` is

`=> lim_(x-> 0^(-)) f(x) =lim_(x-> 0^(-)) (-x) =0`

Similarly, the right hand limit of `f` at `0` is

`=> lim_(x->0^+) f(x) =lim_(x-> 0^+) x=0`

Thus, the left hand limit, right hand limit and the value of the function coincide at `x = 0`. Hence, `f` is continuous at `x = 0`.
Q 3155612564

Check the continuity of the function f given by f (x) = 2x + 3 at x = 1.
Class 12 Chapter 5 Example 1
Solution:

First note that the function is defined at the given point x = 1 and its value is 5.
Then find the limit of the function at x = 1. Clearly

`lim_(x->1) f(x) = lim_(x->1) (2x +3) = 2(1) +3 = 5`

Thus ` lim_(x->1) f(x) = 5 = f (1)`

Hence, f is continuous at x = 1.
Q 3125712661

Examine whether the function f given by `f (x) = x^2` is continuous at x = 0.
Class 12 Chapter 5 Example 2
Solution:

First note that the function is defined at the given point x = 0 and its value is 0.
Then find the limit of the function at x = 0. Clearly

` lim_(x->0) f(x) = lim_(x->0) x^2 = 0^2 =0`

Thus `lim_(x->0) f(x) = 0 = f(0)`

Hence, f is continuous at x = 0.
Q 3185712667

Discuss the continuity of the function f given by f(x) = | x | at x = 0.
Class 12 Chapter 5 Example 3
Solution:

By definition

`f(x) = { tt ( ( -x , text (if) x< 0 ), ( x, text (if) x ge 0 ) )`


Clearly the function is defined at 0 and f (0) = 0. Left hand limit of f at 0 is

` lim_(x->0^-) f(x) = lim_(x->0^-) (-x) = 0`

Similarly, the right hand limit of f at 0 is

`lim_(x->0^+) f(x) = lim_(x->0^+) x= 0`


Thus, the left hand limit, right hand limit and the value of the function coincide at
x = 0. Hence, f is continuous at x = 0.
Q 3115812760

Show that the function f given by

`f(x) = { tt ( ( x^3 +3 , text (if) x ≠ 0 ), (1, text(if) x=0) )`


is not continuous at x = 0.
Class 12 Chapter 5 Example 4
Solution:

The function is defined at x = 0 and its value at x = 0 is 1. When x ≠ 0, the
function is given by a polynomial. Hence,

`lim_(x->0) f(x) = lim_(x->0) (x^3 +3) = 0^3 +3 =3`

Since the limit of f at x = 0 does not coincide with f (0), the function is not continuous
at x = 0. It may be noted that x = 0 is the only point of discontinuity for this function.
Q 3135812762

Check the points where the constant function f (x) = k is continuous.
Class 12 Chapter 5 Example 5
Solution:

The function is defined at all real numbers and by definition, its value at any
real number equals k. Let c be any real number. Then

`lim_(x->c) f(x) = lim_(x->c) k = k`

Since `f (c) = k = lim_(x->c) f(x)` for any real number c, the function f is continuous at every real number.
Q 3175812766

Prove that the identity function on real numbers given by` f (x) = x` is
continuous at every real number.
Class 12 Chapter 5 Example 6
Solution:

The function is clearly defined at every point and f (c) = c for every real
number c. Also,

`lim_(x->c) f(x) = lim_(x->c) x = c`


Thus ` lim_(x->c) f(x) = c = f(c)` and hence the function is continuous at every real number.

Having defined continuity of a function at a given point, now we make a natural
extension of this definition to discuss continuity of a function.

Continuity at an interval :

`\color{green} ✍️` A real function `f` is said to be continuous if it is continuous at every point in the domain of `f`.

`\color{green} ✍️` Suppose `f` is a function defined on a closed interval `[a, b]`, then for `f` to be continuous, it needs to be continuous at every point in `[a, b]` including the end points `a` and `b`.

`=>` Continuity of `f` at a means `color{green}{lim_(x-> a^+) f(x) =f(a)}`

`=>` and continuity of `f` at `b` means `color{green}{lim_(x-> b^(-)) f(x) =f(b)}`

`\color{green} ✍️` Observe that only `lim_(x-> a^(-))` and ` lim_(x-> b^(+))` do not make sense. As a consequence of this definition, if f is defined only at one point, it is continuous there, i.e., if the domain of `f` is a singleton, `f` is a continuous function.
Q 3115012860

Is the function defined by f (x) = | x |, a continuous function?
Class 12 Chapter 5 Example 7
Solution:

We may rewrite f as

`f(x) = { tt ( ( -x , text (if) x < 0 ), ( x , text (if) x ge 0 ) )`

By Example 3, we know that f is continuous at x = 0.
Let c be a real number such that c < 0. Then f (c) = – c. Also

`lim_(x->c) f(x) = lim_(x->c) (-x) = -c` (Why?)

Since` lim_(x->c) f(x) = f(c) , f ` is continuous at all negative real numbers.


Now, let c be a real number such that c > 0. Then f (c) = c. Also

`lim_(x->c) f(x) = lim_(x->c) x= c` (why ? )

Since `lim_(x->c ) f (x ) = f(c), f` is continuous at all positive real numbers. Hence, f
is continuous at all points.
Q 3145012863

Discuss the continuity of the function f given by `f (x) = x^3 + x^2 – 1` .
Class 12 Chapter 5 Example 8
Solution:

Clearly f is defined at every real number c and its value at c is `c^3 + c^2 – 1`. We
also know that

`lim_(x->c) f(x) = lim_(x->c) (x^3 + x^2 -1) = c^3 + c^2 -1`

Thus ` lim_(x->c) f(x) = f(c) , and hence f is continuous at every real number. This means
f is a continuous function.
 
SiteLock