𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐞𝐝 𝐒𝐭𝐫𝐮𝐜𝐭𝐮𝐫𝐞𝐬 :
In solids constituent particles are closed-packed, leaving the minimum vacant space. Constituent particles are considered as identical hard spheres.
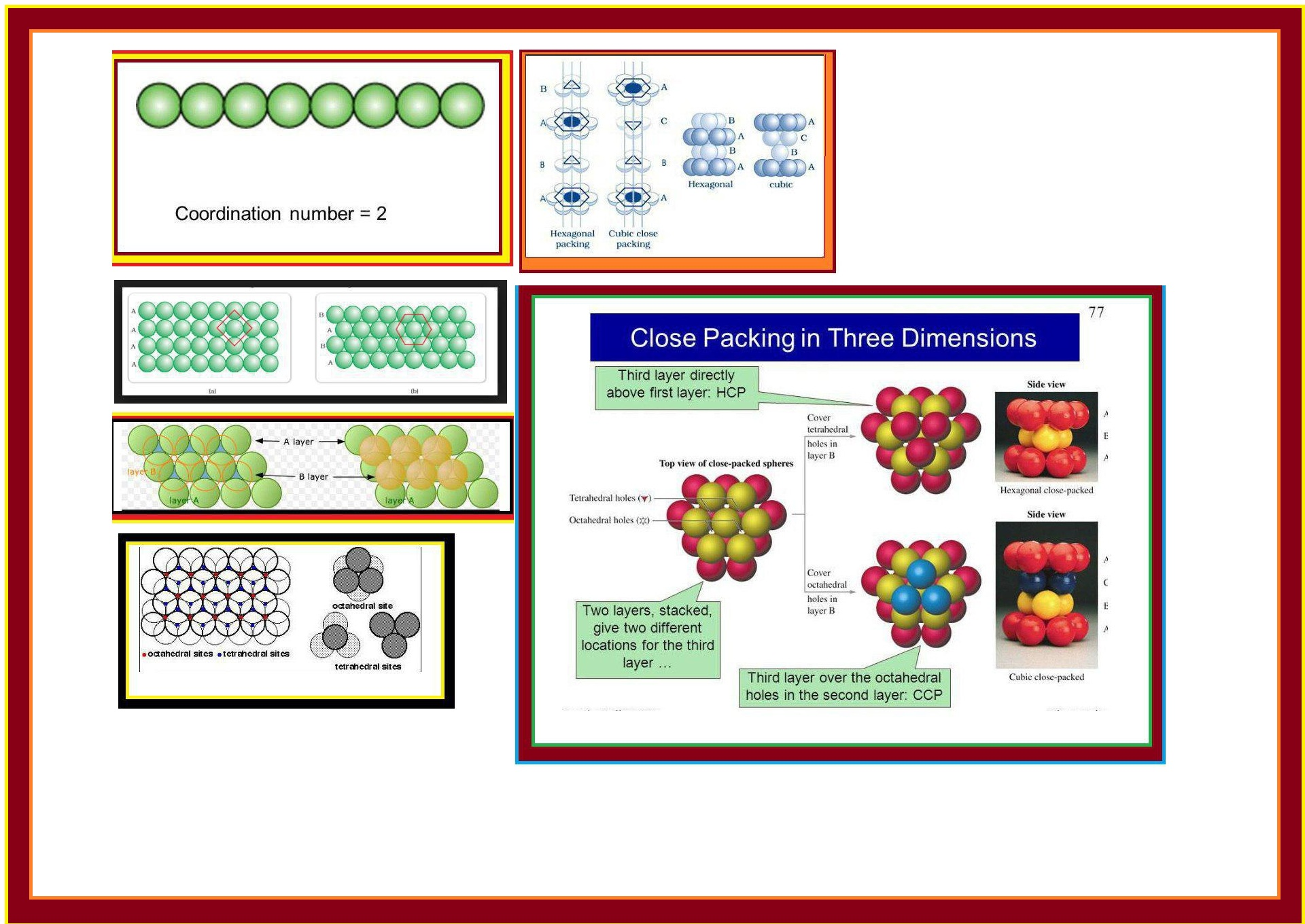
(𝐢) 𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐢𝐧𝐠 𝐢𝐧 𝐎𝐧𝐞 𝐃𝐢𝐦𝐞𝐧𝐬𝐢𝐨𝐧 (𝟏 𝐃) : There is only one way of arranging spheres in one dimensional. We can arrange them in a row and touching each other (Fig 1.13).
The number of nearest neighbours of a particle is called its co-ordination number. Thus in 1-D close packed arrangement the co-ordination number is `2`.
`color{purple}(✓✓)color{purple} " DEFINITION ALERT"`
The number of nearest neighbouring particles around a specific particle in a given crystalline substance is called as coordination number of that crystalline substance
(𝐢𝐢) 𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐢𝐧𝐠 𝐢𝐧 𝐓𝐰𝐨 𝐃𝐢𝐦𝐞𝐧𝐬𝐢𝐨𝐧𝐬 (𝟐 𝐃) : Two dimensional close packed structure can be generated in two different ways.
(a) One row may be placed over other row in a way that the spheres of the one row are exactly above those of the other row and doing so we get `A A A.....` type of arrangement as shown in fig 1.14 (a).
`=>` In this each sphere is in contact with `4` of its neighbours.
Therefore, its co-ordination number is `4`. It is also called `text(square close packing in two dimensions)`.
(b) In this second row may be placed above the first one in a staggered manner such that its sphere fit in the depression of the first row. This is `ABAB.....` type arrangement.
In this arrangement there is less free space. Each sphere is in contact with `6` neighbouring spheres.
Therefore, its co-ordination number is `6`. This is also called `text(two dimensional hexagonal close-packing)` (Fig 1.14 b).
There are some voids (empty spaces) in this arrangement. These are triangular in shape. These are of two types i.e. upward triangular voids and downwards triangular voids.
(𝐢𝐢𝐢) 𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐢𝐧𝐠 𝐢𝐧 𝐓𝐡𝐫𝐞𝐞 𝐃𝐢𝐦𝐞𝐧𝐬𝐢𝐨𝐧𝐬 (𝟑𝐃) : These are obtained by stacking two dimensional layers one above the other. Types of 3-D close-packed structure obtained is :
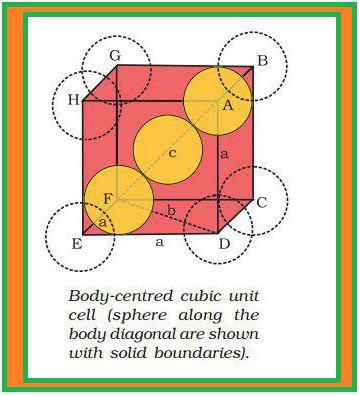
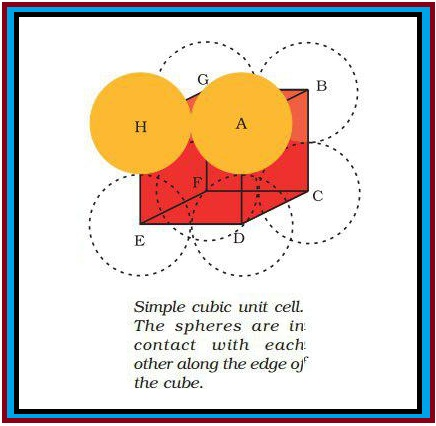
(a) Three Dimensional Close Packing from Two Dimensional Square Closed Packed Layers : The second layer is placed over the first layer such that the spheres of the upper layer are exactly above those of the first layer (Fig 1.15). This is `A A A......` type pattern. This is simple cubic lattice and its unit cell is the primitive cubic unit cell (Fig. 1.9).
(b) Three Dimensional Close Packing from Two Dimensional Hexagonal Close Packed Layers : This is done in following ways :
(A) Placing Second Layer over the First Layer : Second layer is placed above the first layer in a way that it covers the depressions of the first layers. It is observed that all triangular voids are not covered (Fig 1.16).
Wherever a sphere of the second layer is above the void of the first layer (or vice-versa), a void is formed called `text(tetrahedral void)` because a tetrahedron is formed when the centres of these four spheres are joined. It is marked as `T` in Fig 1.16. See Fig 1.17 also.
At some places the triangular voids in the second layer are above the triangular voids in the first layer and voids formed by this are called `text(octahedral voids)` because these voids are surrounded by six sphere. This is marked as `O` in Fig 1.16. See Fig 1.17 also.
The number of these voids depends upon the number of close packed spheres.
Let the number of close packed spheres be `N`, then :
The number of octahedral voids generated `= N`
The number of tetrahedral voids generated `= 2N`
(B) Placing Third Layer over the Second Layer : In this case, there are two possibilities.
(𝐈) 𝐂𝐨𝐯𝐞𝐫𝐢𝐧𝐠 𝐓𝐞𝐭𝐫𝐚𝐡𝐞𝐝𝐫𝐚𝐥 𝐕𝐨𝐢𝐝𝐬 : Tetrahedral voids of the second layer are covered by the spheres of third layer. So, the spheres of third layer are exactly aligned with those of the first layer. So, we get `ABAB......` pattern. This structure is called `text[hexagonal close packed (hcp)]` structure (Fig 1.18). e.g. `Zn` and `Mg`.
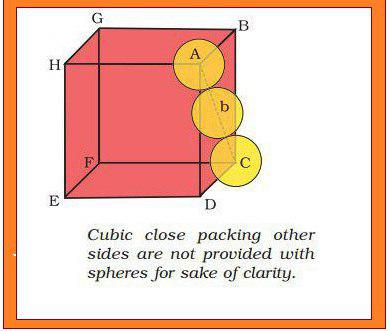
(𝐈𝐈) 𝐂𝐨𝐯𝐞𝐫𝐢𝐧𝐠 𝐎𝐜𝐭𝐚𝐡𝐞𝐝𝐫𝐚𝐥 𝐕𝐨𝐢𝐝𝐬 : In this case, third layer is placed in such a way that its spheres cover the octahedral voids. In this way, the spheres of the third layer are not aligned with spheres of either first or third layer. So, `ABCABC.........` pattern is obtained. This structure is called `text[cubic close packed (ccp)]` or `text[face-centred cubic (fcc)]` structure. e.g `Ag` and `Cu`.
Note : (x) Both these types of packing are highly efficient.
(y) `74%` space is filled.
(z) Each sphere is in contact with twelve spheres. So, co-ordination number is `12`.
(𝐢) 𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐢𝐧𝐠 𝐢𝐧 𝐎𝐧𝐞 𝐃𝐢𝐦𝐞𝐧𝐬𝐢𝐨𝐧 (𝟏 𝐃) : There is only one way of arranging spheres in one dimensional. We can arrange them in a row and touching each other (Fig 1.13).
The number of nearest neighbours of a particle is called its co-ordination number. Thus in 1-D close packed arrangement the co-ordination number is `2`.
`color{purple}(✓✓)color{purple} " DEFINITION ALERT"`
The number of nearest neighbouring particles around a specific particle in a given crystalline substance is called as coordination number of that crystalline substance
(𝐢𝐢) 𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐢𝐧𝐠 𝐢𝐧 𝐓𝐰𝐨 𝐃𝐢𝐦𝐞𝐧𝐬𝐢𝐨𝐧𝐬 (𝟐 𝐃) : Two dimensional close packed structure can be generated in two different ways.
(a) One row may be placed over other row in a way that the spheres of the one row are exactly above those of the other row and doing so we get `A A A.....` type of arrangement as shown in fig 1.14 (a).
`=>` In this each sphere is in contact with `4` of its neighbours.
Therefore, its co-ordination number is `4`. It is also called `text(square close packing in two dimensions)`.
(b) In this second row may be placed above the first one in a staggered manner such that its sphere fit in the depression of the first row. This is `ABAB.....` type arrangement.
In this arrangement there is less free space. Each sphere is in contact with `6` neighbouring spheres.
Therefore, its co-ordination number is `6`. This is also called `text(two dimensional hexagonal close-packing)` (Fig 1.14 b).
There are some voids (empty spaces) in this arrangement. These are triangular in shape. These are of two types i.e. upward triangular voids and downwards triangular voids.
(𝐢𝐢𝐢) 𝐂𝐥𝐨𝐬𝐞 𝐏𝐚𝐜𝐤𝐢𝐧𝐠 𝐢𝐧 𝐓𝐡𝐫𝐞𝐞 𝐃𝐢𝐦𝐞𝐧𝐬𝐢𝐨𝐧𝐬 (𝟑𝐃) : These are obtained by stacking two dimensional layers one above the other. Types of 3-D close-packed structure obtained is :
(a) Three Dimensional Close Packing from Two Dimensional Square Closed Packed Layers : The second layer is placed over the first layer such that the spheres of the upper layer are exactly above those of the first layer (Fig 1.15). This is `A A A......` type pattern. This is simple cubic lattice and its unit cell is the primitive cubic unit cell (Fig. 1.9).
(b) Three Dimensional Close Packing from Two Dimensional Hexagonal Close Packed Layers : This is done in following ways :
(A) Placing Second Layer over the First Layer : Second layer is placed above the first layer in a way that it covers the depressions of the first layers. It is observed that all triangular voids are not covered (Fig 1.16).
Wherever a sphere of the second layer is above the void of the first layer (or vice-versa), a void is formed called `text(tetrahedral void)` because a tetrahedron is formed when the centres of these four spheres are joined. It is marked as `T` in Fig 1.16. See Fig 1.17 also.
At some places the triangular voids in the second layer are above the triangular voids in the first layer and voids formed by this are called `text(octahedral voids)` because these voids are surrounded by six sphere. This is marked as `O` in Fig 1.16. See Fig 1.17 also.
The number of these voids depends upon the number of close packed spheres.
Let the number of close packed spheres be `N`, then :
The number of octahedral voids generated `= N`
The number of tetrahedral voids generated `= 2N`
(B) Placing Third Layer over the Second Layer : In this case, there are two possibilities.
(𝐈) 𝐂𝐨𝐯𝐞𝐫𝐢𝐧𝐠 𝐓𝐞𝐭𝐫𝐚𝐡𝐞𝐝𝐫𝐚𝐥 𝐕𝐨𝐢𝐝𝐬 : Tetrahedral voids of the second layer are covered by the spheres of third layer. So, the spheres of third layer are exactly aligned with those of the first layer. So, we get `ABAB......` pattern. This structure is called `text[hexagonal close packed (hcp)]` structure (Fig 1.18). e.g. `Zn` and `Mg`.
(𝐈𝐈) 𝐂𝐨𝐯𝐞𝐫𝐢𝐧𝐠 𝐎𝐜𝐭𝐚𝐡𝐞𝐝𝐫𝐚𝐥 𝐕𝐨𝐢𝐝𝐬 : In this case, third layer is placed in such a way that its spheres cover the octahedral voids. In this way, the spheres of the third layer are not aligned with spheres of either first or third layer. So, `ABCABC.........` pattern is obtained. This structure is called `text[cubic close packed (ccp)]` or `text[face-centred cubic (fcc)]` structure. e.g `Ag` and `Cu`.
Note : (x) Both these types of packing are highly efficient.
(y) `74%` space is filled.
(z) Each sphere is in contact with twelve spheres. So, co-ordination number is `12`.