`\color{blue}✍️` An electric dipole is a pair of equal and opposite point charges `q` and `–q`, separated by a distance `2a`.

`\color{blue}✍️` The line connecting the two charges defines a direction in space.

`\color{blue}✍️` The direction from `–q` to `q` is said to be the direction of the dipole.

`\color{blue}✍️` The mid-point of locations of –q and q is called the `centre` of the dipole.

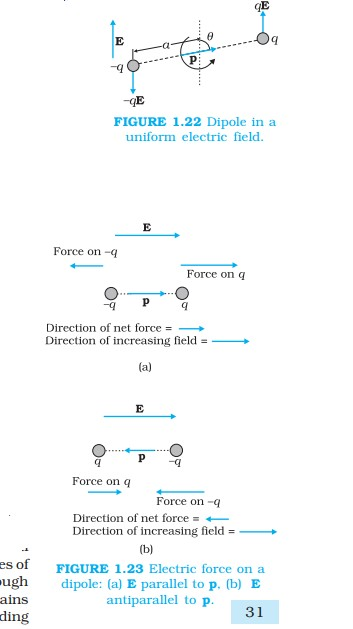
`\color{blue}✍️` The total charge of the electric dipole is obviously zero. This does not mean that the field of the electric dipole is zero. Since the charge `q` and `–q` are separated by some distance, the electric fields due to them, when added, do not exactly cancel out. However, at distances much larger than the separation of the two charges forming a dipole `(r > > 2a)`, the fields due to`q` and ` –q` nearly cancel out. The electric field due to a dipole therefore falls off, at large distance, faster than like` 1/r^2` .

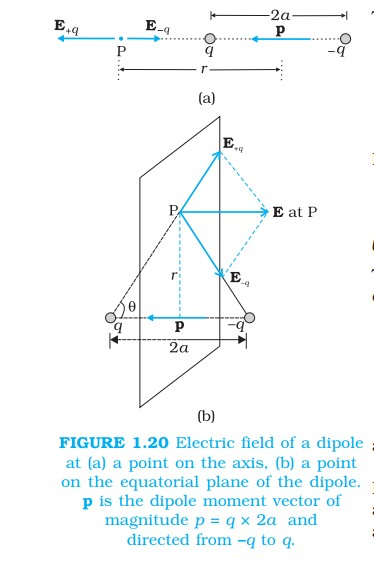
The electric field of the pair of charges (`–q` and `q`) at any point in space can be found out from Coulomb’s law and the superposition principle.

`\color{blue} ✍️` The results are simple for the following two cases:

`color{blue} {(i)}` When the point is on the dipole axis,

`color{blue} {(ii)}` When it is in the equatorial plane of the dipole, i.e., on a plane perpendicular to the dipole axis through its centre.

`\color{blue} ✍️`The electric field at any general point P is obtained by adding the electric fields `E_(–q)` due to the charge `–q` and `E_(+q)` due to the charge `q`, by the parallelogram law of vectors.

`color{blue}{(i)}` For points on the axis
Let the point `P` be at `r` from the centre of the dipole on the side of the charge `q`. Then

`E_(-q) =- q/(4πε_0(r+a)^2)hat p`

where `hat p` is the unit vector along the dipole axis (from `–q` to `q`) . Also

`E_(+q) = q/(4πε_0(r-a)^2)hat p`

The total field at `P` is

`E =E_(+q)+E_(-q)= q/(4πε_0)[1/(r-a)^2-1/(r+a)^2]hat p`

`= q/(4πε_0)(4ar)/(r^2-a^2)^2`

`\color{blue} ✍️`For `r > > a`

`E=(4qa)/(4πε_0r^3) hat p`

`color{blue}{(ii)}` For points on the equatorial plane :

The magnitudes of the electric fields due to the two charges `+q` and `–q` are given by

`E_(+q) = q/(4πε_0(r-a)^2)hat p` and

`E_(-q) =- q/(4πε_0(r+a)^2)hat p`

are equal.

`color{blue}●`The components normal to the dipole axis cancel away.

`color{blue}●`The components along the dipole axis add up. The total electric field is opposite to `hat p`. We have

`E = - (E_(+q) + E_(–q) ) cosθ hat p`

=`- (2qa)/(4πε_0(r^2+a^2)^(3/2)) hat p`

At large distances `(r > > a)`, this reduces

`E=- (2qa)/(4πε_0r^3) hatp`

`color{blue}●` The dipole field at large distances does not involve `q` and` a `separately;

`color{blue}●` It depends on the product `qa`.

The dipole moment vector `p` of an electric dipole is defined by `p = q × 2a hat p`

`color{blue}●` It is a vector whose magnitude is charge `q` times the separation `2a`.

`color{blue}●` The direction is along the line from `–q` to `q`. In terms of `p`.

`color{blue}●` The electric field of a dipole at large distances takes simple forms :

`color{blue}{1.}` At a point on the dipole axis:

`E=(2P)/(4πε_0r^3)` (r >> a)

`color{blue}{2.}` At a point on the equatorial plane

`E=(-P)/(4πε_0r^3)` (r >> a)

`color{blue}●` The dipole field at large distances falls off not as `1/r^2` but as `1/r^3`.

`color{blue}●` The magnitude and the direction of the dipole field depends not only on the distance r but also on the angle between the position vector r and the dipole moment p.

`color{blue}●` When the dipole size 2 a approaches zero, the charge q approaches infinity in such a way that the product `p = q × 2 a` is finite. Such a dipole is referred to as a point dipole.

`color{blue}{1.}` In most molecules, the centres of positive charges and of negative charges lie at the same place. Therefore, their dipole moment is zero. `CO_2 ` and `CH_4` are of this type of molecules. However, they develop a dipole moment when an electric field is applied.

`color{blue}{2.}` In some molecules, the centres of negative charges and of positive charges do not coincide. Therefore they have a permanent electric dipole moment, even in the absence of an electric field. Such molecules are called polar molecules. Water molecules, `H_2O`, is an example of this type.

Consider a permanent dipole of dipole moment p in a uniform external field E. There is a force `qE` on `q` and a force `–qE` on `–q`. The net force on the dipole is zero, since E is uniform. When the net force is zero, the torque is independent of the origin. Its magnitude equals the magnitude of each force multiplied by the arm of the couple.


Magnitude of torque `= q E × 2 a sinθ`

`= 2 q a E sinθ`

Its direction is normal to the plane of the paper, coming out of it.

The magnitude of `p × E ` is also `p E sinθ` and its direction is normal to the paper, coming out of it.
Thus,

`τ = p × E `

This torque will tend to align the dipole with the field `E`. When `p` is aligned with `E`, the torque is zero.

If the field is not uniform, In that case, the net force will evidently be non-zero.

The force depends on the orientation of `p` with respect to `E`.

A surface charge density `σ` at the area element by

`σ=(ΔQ)/(ΔS)`

`σ=` the surface charge density.

`ΔQ=` charge contain in area element.

The units for ` σ` are `C/m^2` .

The linear charge density `λ` of a wire is defined by

`λ = (ΔQ)/(Δl)`

`Δl =` a small line element of wire

`ΔQ` is the charge contained in that line element.
The units for `λ` are `C/m` .

The volume charge density (sometimes simply called charge density)
is defined in a similar manner:

`ρ=(ΔQ)/(ΔV)`

`ΔQ` is the charge included in the small volume element `ΔV` that includes a large number of microscopic charged
constituents.

The units for `ρ` are` C/m^3` .

Consider any general point `P` with position vector `R` . Electric field due to the charge `ρΔV` is given by Coulomb’s law :

`ΔE=1/(4πε_0)(ρΔV)/r'^2hatr' `

where ` r′ ` = distance between the charge element and `P`

`hatr′` = unit vector in the direction from the charge element to `P`.

let us consider the total flux through a sphere of radius `r`, which encloses a point charge `q` at its centre. Divide the sphere into small area elements, as shown in Fig. below


Electric flux through a closed surface `S`

= `q/ε_0`

`q =` total charge enclosed by `S`.

Let us consider the total flux through a sphere of radius r, which encloses a point charge q at its centre. Divide the sphere into small area elements, as shown in

The flux through an area element `ΔS` is

`Δφ=E.ΔS=q/(4πε_0r^2)r. Δ S`

The unit vector `hat r` is along the radius vector from the centre to the area element. Now, since the normal to a sphere at every point is along the radius vector at that point, the area element `ΔS` and `hat r` have the same direction. Therefore,

`Δφ=q/(4πε_0r^2)ΔS`

The total flux through the sphere is obtained by adding up flux through all the different area elements:

`φ=sum_(allΔs )q/(4πε_0r^2 )ΔS`




Since each area element of the sphere is at the same distance r from the charge,

`φ=q/(4πε_0r^2 )sum_(allΔs )ΔS=q/(4πε_0r^2 )S`

Now `S`, the total area of the sphere, equals `4πr^ 2` . Thus,

`φ=(q/(4πε_0r^2 ))*(4πr^2) =q/ε_0`

Here the electric field is uniform and we are considering a closed cylindrical surface, with its axis parallel to the uniform field `E`. The total
flux `φ` through the surface is `φ = φ_1 + φ_2 + φ_3,` where `φ_1` and `φ_2` represent the flux through the surfaces `1` and `2` of the cylinder and `φ_3` is the flux through the curved cylindrical part of the closed surface. Now the normal to the surface `3` at every point is perpendicular to `E`, so by definition of flux, `φ_3 = 0`. Further, the outward normal to `2` is along `E` while the outward normal to `1` is opposite to `E`.

Therefore,
`φ_1 = –E S_1, φ_2 = +E S_2`

`S_1 = S_2 = S`

where `S` is the area of circular cross-section. Thus, the total flux is zero, as expected by Gauss’s law. Thus, whenever you find that the net electric flux through a closed surface is zero, we conclude that the total charge contained in the closed surface is zero.

`\color{green} ✍️ \color{green} \mathbf(KEY \ CONCEPT)`
The great significance of Gauss’s law , is that it is true in general, and not only for the simple cases we have considered above. Let
us note some important points regarding this law:

`color{blue}{(i)}` Gauss’s law is true for any closed surface, no matter what its shape or size.

`color{blue}{(ii)}` The term `q` on the right side of Gauss’s law , includes the sum of all charges enclosed by the surface. The charges may be located anywhere inside the surface.

`color{blue}{(iii)}` When the surface is so chosen that there are some charges inside and some outside, the electric field is due to all the charges, both inside and outside `S`. The term `q` on the right side of Gauss’s law, however, represents only the total charge inside `S`.

`color{blue}{(iv)}` The surface that we choose for the application of Gauss’s law is called the Gaussian surface. You may choose any Gaussian surface and apply Gauss’s law. However, take care not to let the Gaussian surface pass through any discrete charge. This is because electric field due to a system of discrete charges is not well defined at the location of any charge .However, the Gaussian surface can pass through a continuous charge distribution.

`color{blue}{(v)}` Gauss’s law is often useful towards a much easier calculation of the electrostatic field when the system has some symmetry. This is facilitated by the choice of a suitable Gaussian surface.

`color{blue}{(vi)}` Finally, Gauss’s law is based on the inverse square dependence on distance contained in the Coulomb’s law. Any violation of Gauss’s law will indicate departure from the inverse square law.

`color{blue}{1.} color{blue}bbul{" Field due to an infinitely long straight uniformly charged wire:"}`

Imagine a cylindrical Gaussian surface .Since the field is everywhere radial, flux through the two ends of the cylindrical Gaussian surface is zero.


At the cylindrical part of the surface, `E` is normal to the surface at every point, and its magnitude is constant, since it depends only on `r`. The surface are of the curved part is `2πrl`, where l is the length of the cylinder. Flux through the Gaussian surface

`=` flux through the curved cylindrical part of the surface

`= E × 2πrl`

The surface includes charge equal to `λ_l` . Gauss’s law then gives

` E × 2πrl = (λl)/(ε_0)`

i.e., ` E =λ/(2πε_0r)`

Vectorially, `E` at any point is given by:
` E =λ/(2πε_0r) hat n `

where `hat n` is the radial unit vector in the plane normal to the wire passing through the point. `E` is directed outward if `λ` is positive and inward if `λ` is negative.

`2.color{green}bbul{" Field due to a uniformly charged infinite plane shee"}`

Let `σ` be the uniform surface charge density of an infinite plane sheet We can take the Gaussian surface to be a rectangular paralleleopiped of cross sectional area `A`


Therefore, flux `E*ΔS` through both the surfaces are equal and add up. Therefore the net flux through the Gaussian surface is `2 EA`.

The charge enclosed by the closed surface is `σA`.

Therefore by Gauss’s law,

`2 EA = σA/ε_0`

or, `E = σ/2ε_0`

Vectorically,

`E = σ/(2ε_0) hat n`

where `hat n` is a unit vector normal to the plane and going away from it. `E` is directed away from the plate if `σ` is positive and toward the plate if `σ` is negative.

`color{blue}{3.} color{blue}bbul{"Field due to a uniformly charged thin spherical shell"}`

Let `σ` be the uniform surface charge density of a thin spherical shell of radius `R`. The field at any point `P`, outside or inside, can depend only on `r` .


`color{blue}{(i)}` Field outside the shell :

Consider a point `P` outside the shell with radius vector `r`. To calculate `E` at `P`, we take the Gaussian surface to be a sphere of radius `r` and with centre `O`, passing through `P`. All points on this sphere are equivalent relative to the given charged configuration. The electric field at each point of the Gaussian surface, therefore, has the same magnitude E and is along the radius vector at each point. Thus, `E` and `ΔS` at every point are parallel and the flux through each element is `E ΔS`. Summing over all `ΔS`, the flux through the Gaussian surface is

`E × 4 π r ^2`. The charge enclosed is `σ × 4 π R^2`. By Gauss’s law

`E × 4 π r^ 2 = σ/ε_0(4πR^2)`

Or, `E = (σR^2)/(ε_0r^2) =q/(4πε_0r^2)`

where ` q = 4 π R^2 σ` is the total charge on the spherical shell.

Vectorially, `E =q/(4πε_0r^2) hat r`

The electric field is directed outward if `q > 0` and inward if `q < 0`. This, however, is exactly the field produced by a charge `q` placed at the centre `O`. Thus for points outside the shell, the field due to a uniformly charged shell is as if the entire charge of the shell is concentrated at its centre.

`color{blue}{(ii)}` Field inside the shell :

The point `P` is inside the shell. The Gaussian surface is again a sphere through `P` centred at `O`.The flux through the Gaussian surface, calculated as before, is

` E × 4 π r^2`.

However, in this case, the Gaussian surface encloses no charge. Gauss’s law then gives

`E × 4 π r^2 = 0`
i.e., `E = 0" " (r < R )`

that is, the field due to a uniformly charged thin shell is zero at all points inside the shell*. This important result is a direct consequence of Gauss’s law which follows from Coulomb’s law. The experimental verification of this result confirms the` 1/r^2 ` dependence in Coulomb’s law.