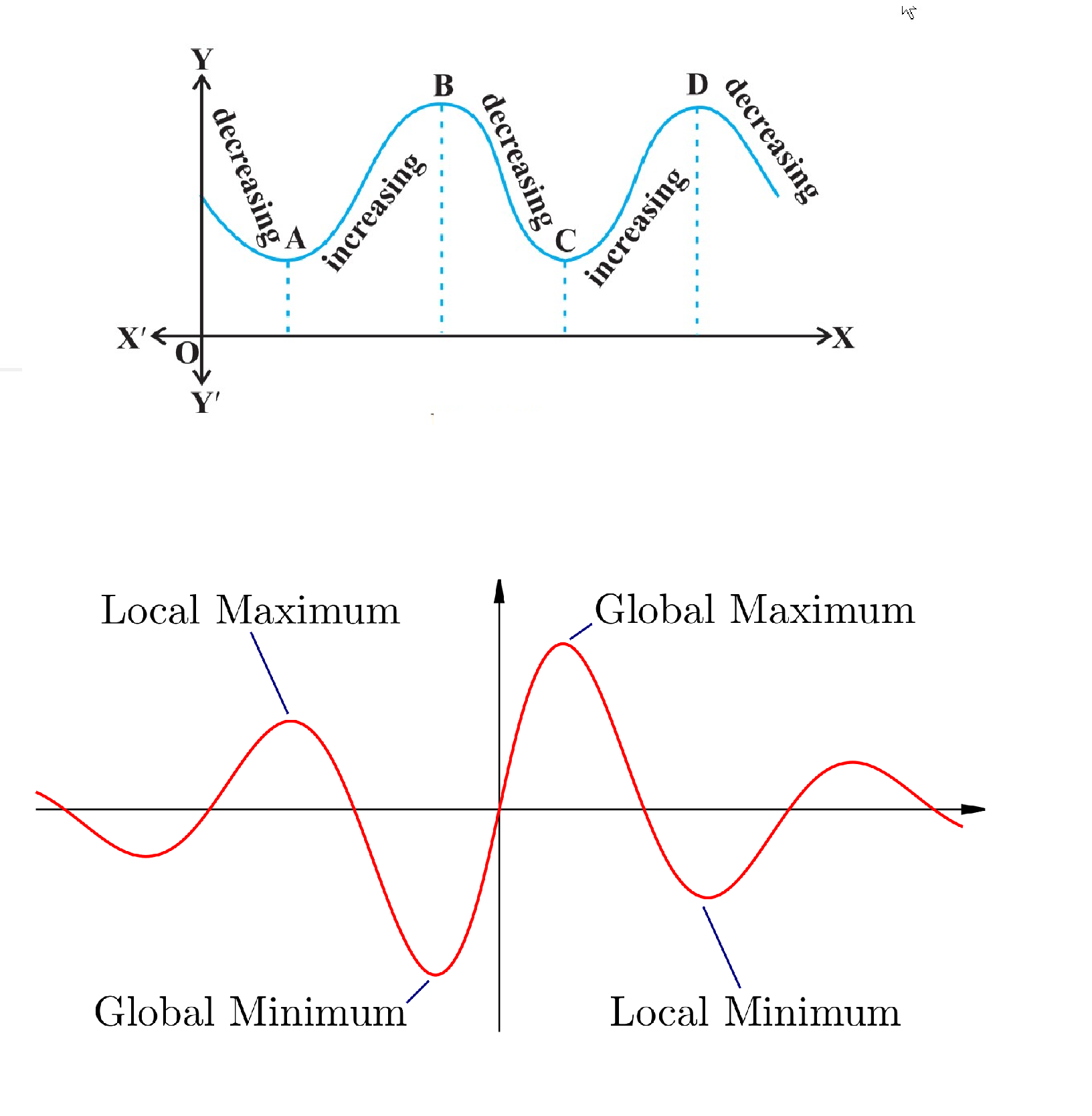
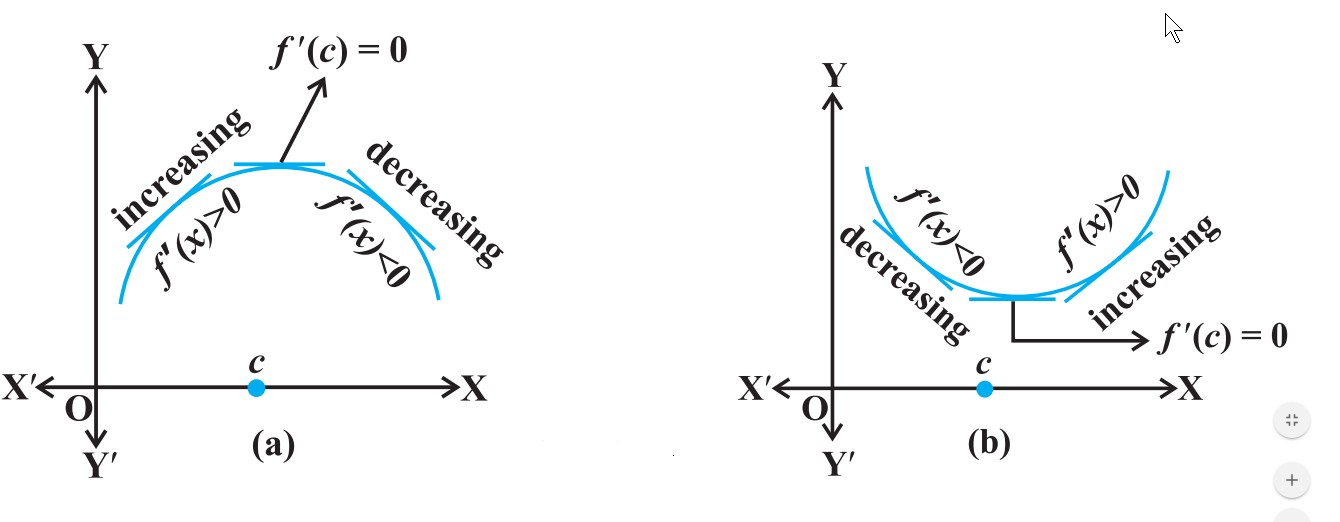
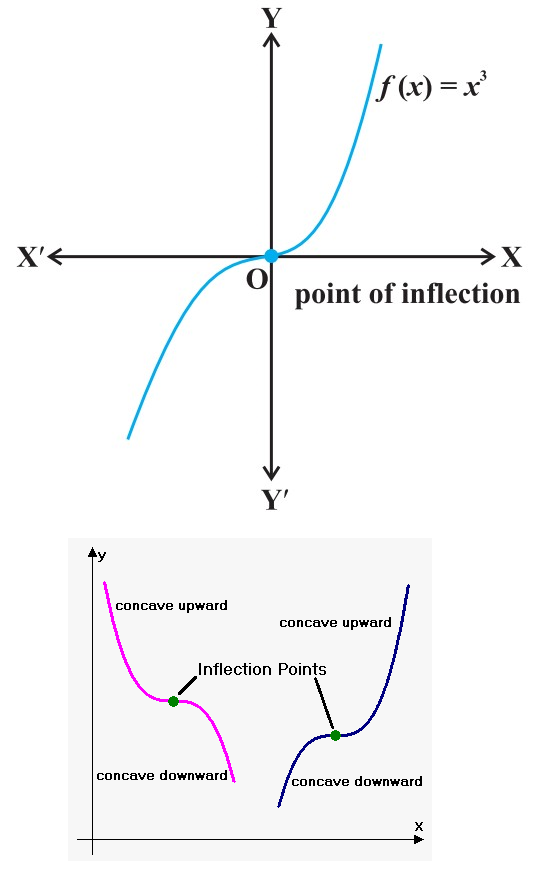
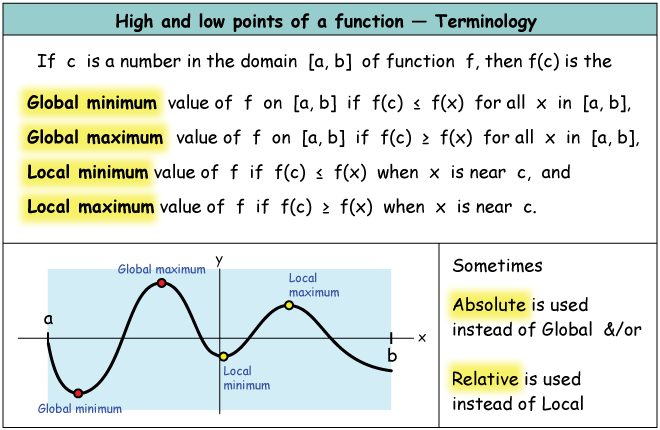
Maxima and Minima
`\color{green} ✍️` Let f be a function defined on an interval `I.`
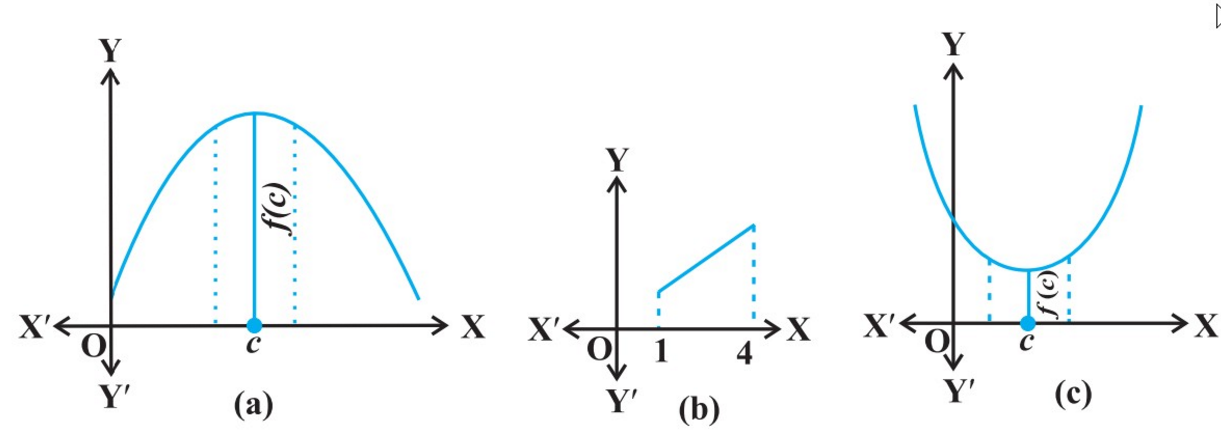
(a) Then `f` is said to have a maximum value in `I,` if there exists a point `c` in `I` such that
`color{green}{f (c) ≥ f (x)}` , for all x ∈ I.
`=>` The number `f(c)` is called the maximum value of `f` in `I` and the point `c` is called a point of maximum value of `f` in `I.`
(b) `f` is said to have a minimum value in `I,` if there exists a point `c` in `I` such that
`color{green}{f (c) ≤ f (x)}`, for all x ∈ I.
`=>` The number `f(c),` in this case, is called the minimum value of f in `I` and the point `c,` in this case, is called a point of minimum value of f in `I.`
`\color{green} ✍️` (c) `f` is said to have an extreme value in `I` if there exists a point c in `I` such that `f (c)` is either a maximum value or a minimum value of `f` in `I.`
`\color{green} ✍️` The number f (c), in this case, is called an extreme value of f in I and the point c is called an extreme point.
(a) Then `f` is said to have a maximum value in `I,` if there exists a point `c` in `I` such that
`color{green}{f (c) ≥ f (x)}` , for all x ∈ I.
`=>` The number `f(c)` is called the maximum value of `f` in `I` and the point `c` is called a point of maximum value of `f` in `I.`
(b) `f` is said to have a minimum value in `I,` if there exists a point `c` in `I` such that
`color{green}{f (c) ≤ f (x)}`, for all x ∈ I.
`=>` The number `f(c),` in this case, is called the minimum value of f in `I` and the point `c,` in this case, is called a point of minimum value of f in `I.`
`\color{green} ✍️` (c) `f` is said to have an extreme value in `I` if there exists a point c in `I` such that `f (c)` is either a maximum value or a minimum value of `f` in `I.`
`\color{green} ✍️` The number f (c), in this case, is called an extreme value of f in I and the point c is called an extreme point.