Area between Two Curves
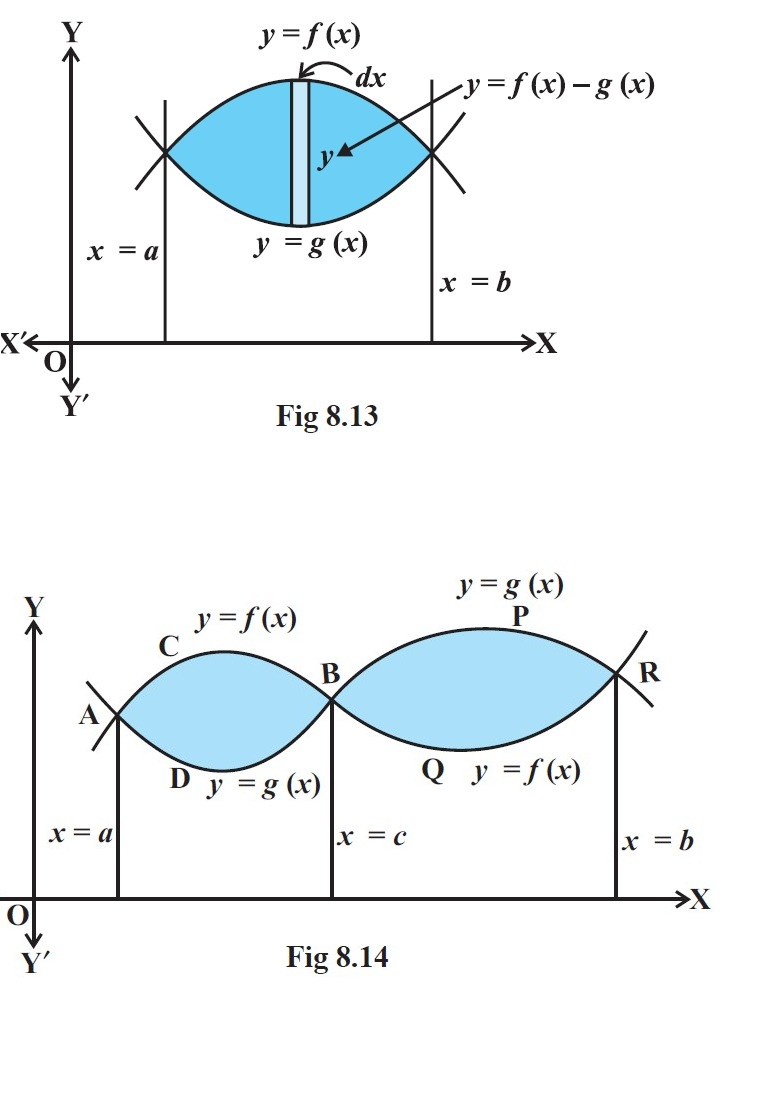
● we are given two curves represented by `y = f (x), y = g (x),` where `f (x) ≥ g(x)` in `[a, b]` as shown in Fig .
● Here the points of intersection of these two curves are given by `x = a` and `x = b` obtained by taking common values of `y` from the given equation of two curves.
● For setting up a formula for the integral, it is convenient to take elementary area in the form of vertical strips. As indicated in the Fig
`f (x) – g(x)` and width `dx` so that the elementary area
`dA = [f (x) – g(x)] dx,` and the total area A can be taken as
`color{orange} {A = int_a^b [ f(x )- g(x )] dx}`
Alternatively,
`A = ["area bounded by" y = f (x), "x-axis and the lines"\ \ x = a, x = b]`
`– ["area bounded by" y = g (x), "x-axis and the lines" \ \ x = a, x = b]`
`color {red} {= int_a^b f(x) dx - int_a^b g(x) dx = int_a^b [ f(x) - g(x) ] dx}` , where `f(x) >= g(x) ` in `[ a,b]`
● If `f (x) ≥ g (x)` in `[a, c]` and `f (x) ≤ g (x)` in `[c, b],` where `a < c < b` as shown in the Fig , then the area of the regions bounded by curves can be written as
Total Area = Area of the region ACBDA + Area of the region BPRQB
`color{red} {= int_a^c [ f(x) - g(x) ] dx + int_c^b [ g(x) - f(x) ] dx}`
● Here the points of intersection of these two curves are given by `x = a` and `x = b` obtained by taking common values of `y` from the given equation of two curves.
● For setting up a formula for the integral, it is convenient to take elementary area in the form of vertical strips. As indicated in the Fig
`f (x) – g(x)` and width `dx` so that the elementary area
`dA = [f (x) – g(x)] dx,` and the total area A can be taken as
`color{orange} {A = int_a^b [ f(x )- g(x )] dx}`
Alternatively,
`A = ["area bounded by" y = f (x), "x-axis and the lines"\ \ x = a, x = b]`
`– ["area bounded by" y = g (x), "x-axis and the lines" \ \ x = a, x = b]`
`color {red} {= int_a^b f(x) dx - int_a^b g(x) dx = int_a^b [ f(x) - g(x) ] dx}` , where `f(x) >= g(x) ` in `[ a,b]`
● If `f (x) ≥ g (x)` in `[a, c]` and `f (x) ≤ g (x)` in `[c, b],` where `a < c < b` as shown in the Fig , then the area of the regions bounded by curves can be written as
Total Area = Area of the region ACBDA + Area of the region BPRQB
`color{red} {= int_a^c [ f(x) - g(x) ] dx + int_c^b [ g(x) - f(x) ] dx}`









