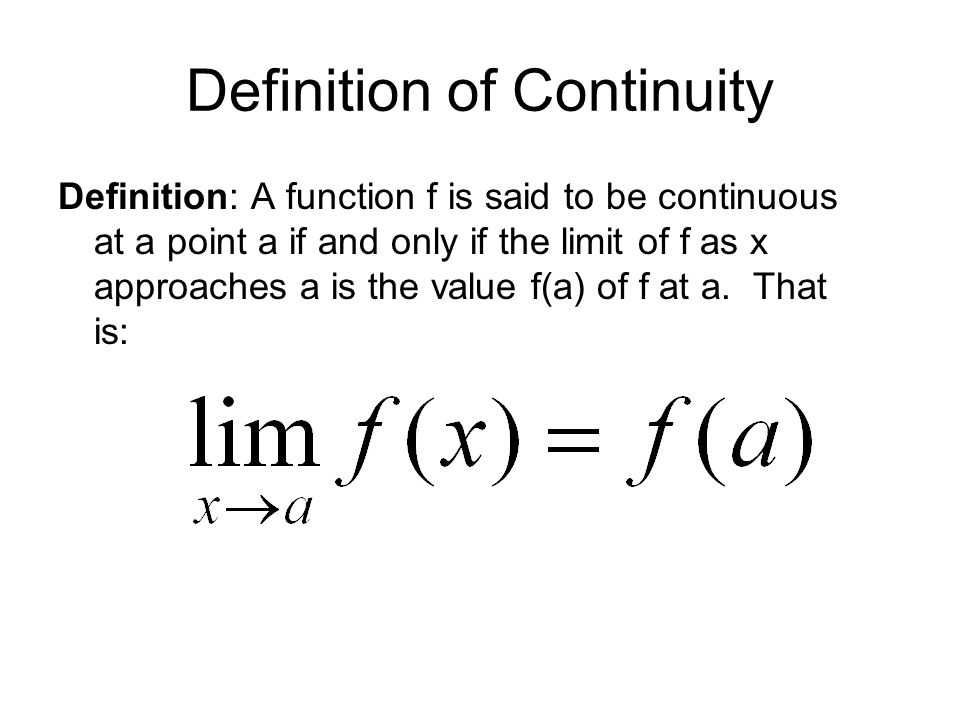
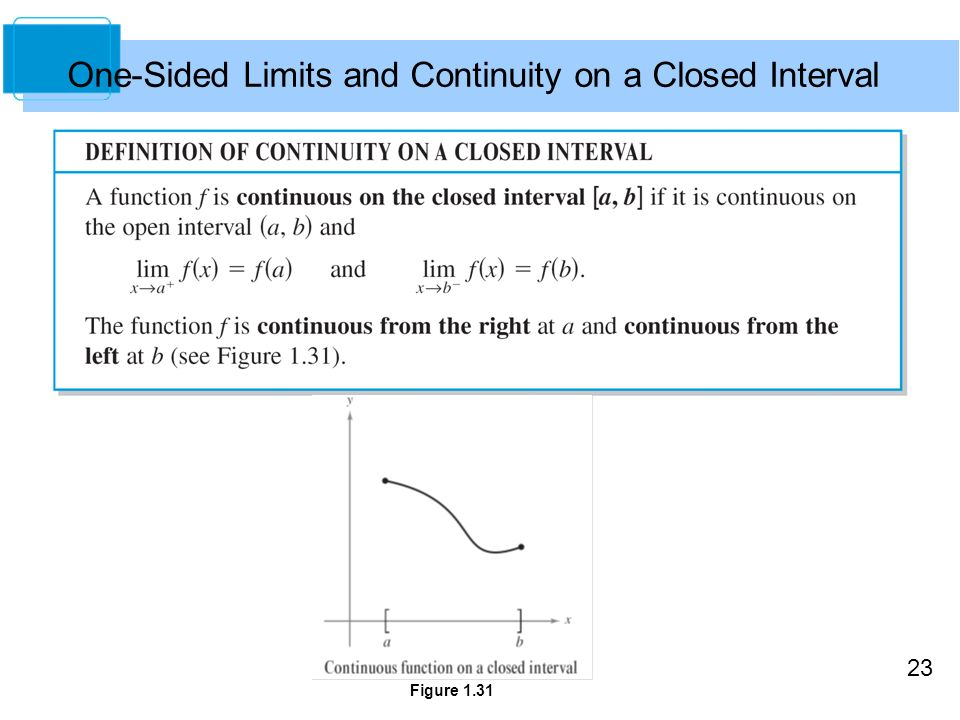
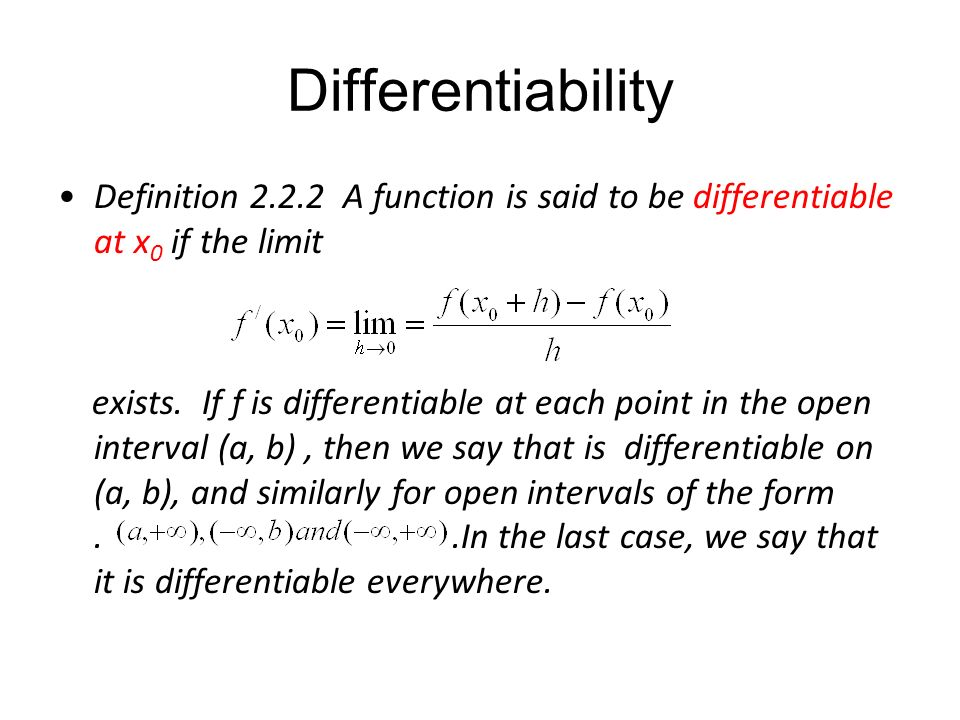
Theorem : If A function f is differentiable at a point c, then it is also continuous at that point.
`color{red}{"Note : So Every differentiable function is continuous at "}`
`color{red}{" that point but that doesn't mean Every continuous "}`
`color{red}{"function is differentiable at the same point."}`
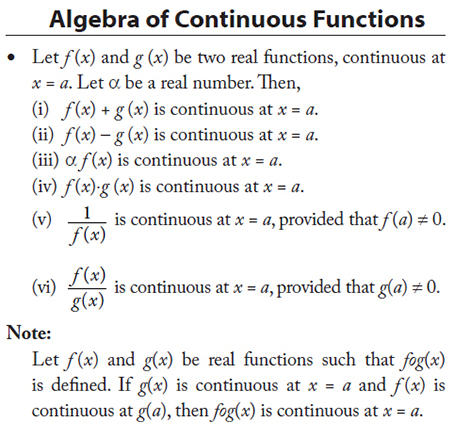
The following rules of algebra of derivatives :
(1) `(u pm v)' = u' pm v'`
(2) `(uv)'= u'v + uv'` (Leibnitz or product rule)
(3) `(u/v)' =((u'v -uv')/v^2)` wherever `v != 0` (Quotient rule).
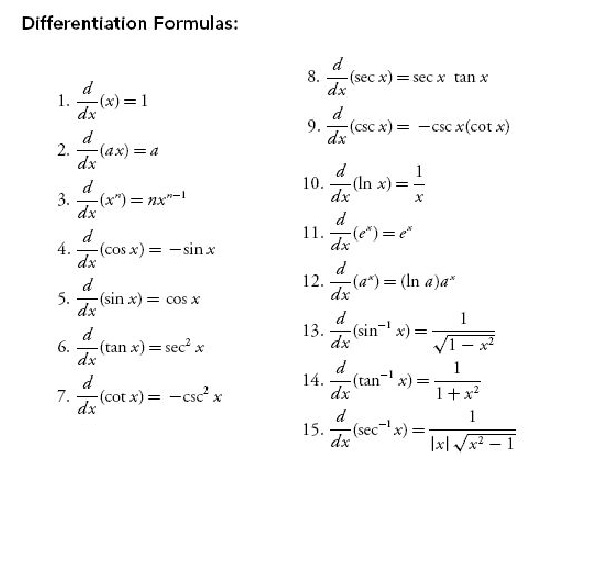
Differentiation formulas list :
Theorem : If A function f is differentiable at a point c, then it is also continuous at that point.
`color{red}{"Note : So Every differentiable function is continuous at "}`
`color{red}{" that point but that doesn't mean Every continuous "}`
`color{red}{"function is differentiable at the same point."}`
The following rules of algebra of derivatives :
(1) `(u pm v)' = u' pm v'`
(2) `(uv)'= u'v + uv'` (Leibnitz or product rule)
(3) `(u/v)' =((u'v -uv')/v^2)` wherever `v != 0` (Quotient rule).
Differentiation formulas list :